Binary Search Tree From Sorted Array Leetcode

Problem: Given an integer array nums where the elements are sorted in ascending order, convert it to a height-balanced binary search tree.

A height-balanced binary tree is a binary tree in which the depth of the two subtrees of every node never differs by more than one.

 Example 1:

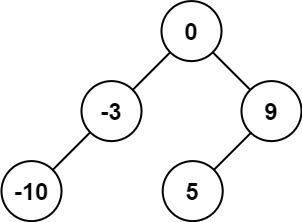
Input: nums = [-10,-3,0,5,9]
Output: [0,-3,9,-10,null,5]
Explanation: [0,-10,5,null,-3,null,9] is also accepted:

Example 2:

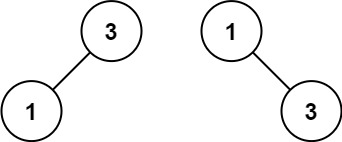
Input: nums = [1,3]
Output: [3,1]
Explanation: [1,3] and [3,1] are both a height-balanced BSTs.

 Constraints:

• 1 <= nums.length <= 104
• -104 <= nums[i] <= 104
• nums is sorted in a strictly increasing order.

This problem is popular in LeetCode and GeeksForGeeks A collection of hundreds of interview questions and solutions are available in our blog at Interview Question

Solution

	/**
	Given a sorted (increasing order) array with unique integer elements, write an algorithm
	to create a binary search tree with minimal height.
	Method1:
	-we find the item at the middle index and make it as a root and make the left half as left subtree and right half as right subtree
	-the process is repeated recursively
	*/
	import java.util.List;
	import java.util.LinkedList;
	import java.util.Queue;
	//a simple representation of Tree
	enum NodeType{sep, reg}
	class TreeNode{
	int val;
	NodeType type;
	TreeNode left;
	TreeNode right;
	TreeNode(int v){
	val = v;
	type = NodeType.reg;//by default it is a regular node
	}
	void setRight(TreeNode r){
	right = r;
	}
	void setLeft(TreeNode l){
	left = l;
	}
	TreeNode getRight(){
	return right;
	}
	TreeNode getLeft(){
	return left;
	}
	}
	public class BinaryTeeFromSortedArray{
	/**
	we iteratively scan the root and its child nodes and add them to the queue and then finally return the linked list
	*/
	public static List<LinkedList<TreeNode>> getListfromBST(TreeNode root){
	if(root == null){
	return null;
	}
	//create a list of linked list to add the nodes at different level
	List<LinkedList<TreeNode>> nodesList = new LinkedList<LinkedList<TreeNode>>();
	//start with root by adding it to the queue
	Queue<TreeNode> nodeQueue = new LinkedList<TreeNode>();
	nodeQueue.add(root);
	LinkedList<TreeNode> tempList = new LinkedList<TreeNode>();
	while(!nodeQueue.isEmpty()){
	//get the first node of this queue and proceed
	TreeNode first = nodeQueue.poll();
	if(nodesList.size()==0){
	nodesList.add(new LinkedList<TreeNode>());
	}
	//add the node to the last linked list
	nodesList.get(nodesList.size()-1).add(first);
	//fill the tempList for this level of nodes
	if(first.left!=null)
	tempList.add(first.left);
	if(first.right!=null)
	tempList.add(first.right);
	//if the queue is already empty, fill it with the entries from templist to start the new level
	if(nodeQueue.isEmpty()){
	while(tempList.size()>0){
	nodeQueue.add(tempList.removeFirst());
	}
	//create a new list to hold the nodes for next level
	nodesList.add(new LinkedList<TreeNode>());
	}
	}
	return nodesList;
	}
	public static TreeNode createMinimalBST(int[] arr, int start, int end){
	if(end<start){
	return null;
	}
	int mid = (start+end)/2;
	TreeNode root = new TreeNode(arr[mid]);
	root.left = createMinimalBST(arr, start, mid-1);
	root.right = createMinimalBST(arr, mid+1, end);
	return root;
	}
	public static void main(String args[]){
	int[] arr = new int[]{1,2,3,4,5,6,7};
	TreeNode root = createMinimalBST(arr, 0, arr.length-1);
	List<LinkedList<TreeNode>> nodesList = getListfromBST(root);
	//now print the nodes of each list
	for(LinkedList<TreeNode> list: nodesList){
	for(TreeNode node:list){
	System.out.print(node.val+" ");
	}
	System.out.println();
	}
	}
	}

view raw BinaryTreeFromSortedArray.java hosted with ❤ by GitHub